FinalProgram

Math Circle Poster and Activity Session

Joint Math Meetings 2013 San Diego CA

Friday, January 11, 2013, 1-4 PM

Organizers:

Philip B. Yasskin, Texas A&M University, yasskin@math.tamu.edu, 979-845-3734

Sam Vandervelde, St. Lawrence University, svandervelde@stlawu.edu, 315-229-5946

Tatiana Shubin, San Jose State University, shubin@math.sjsu.edu, 408-924-5146

James Tanton, St. Mark's School, jamestanton@stmarksschool.org , 508-460-0350

Come join us for the chance to experience a math circle firsthand. Math circles vary widely in format and frequency, but they all bring groups of interested students or teachers together with professional mathematicians to investigate and discover mathematics. Ten math circles from around the country will display a poster describing that circle along with a live activity to try out. These activities are intended to provide ideas for lessons to use at your own circle or school. Activities will restart every 30 minutes.

The session is sponsored by the Special Interest Group of the MAA on Math Circles for Students and Teachers (SIGMAA-MCST) with collaboration by the National Association of Math Circles (NAMC) and the Math Teachers’ Circle Network (MTCN). Each of these organizations will also have an information table. For a schedule of talks as well as abstracts and handouts, please see http://sigmaa.maa.org/mcst/PosterActivitySessions/JMM2013.

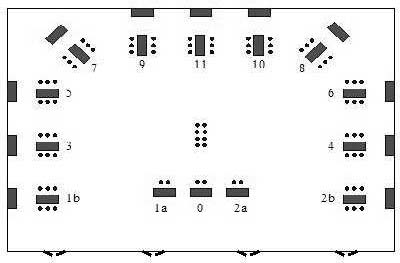
Presentation Time	Table	Table	Table	Table
1:00	4		5	9
1:30	3	11	7	10
2:00	8	10	4	2b
2:30	6	9	3	1b
3:00	1b	5	8	11
3:30	2b	7	6

Table 0: Organization: SIGMAA-MCST http://sigmaa.maa.org/mcst/ Help for Student and Teacher Circles

Activity: Information and Registration Table

Presenters: Sam Vandervelde svandervelde@stlawu.edu --St. Lawrence University

Tatiana Shubin --tatiana.shubin@sjsu.edu --San Jose State University

Philip Yasskin – yasskin@math.tamu.edu --Texas A&M University

James Tanton --jamestanton@stmarksschool.org --St Mark's School

Edward Keppelmann – keppelma@unr.edu --University of Nevada, Reno

Organization Description: SIGMAA MCST (Special Interest Group on Math Circles for Students and Teachers) is sponsoring a poster and activity session to illustrate and celebrate the power and effectiveness of Math Circle work. A Math Circle is broadly defined as a semi-formal, sustained enrichment experience that brings mathematics professionals in direct contact with pre-college students of all ages and/or their teachers. Circles foster passion and excitement for deep mathematics. There are currently over 120 math circles across the nation.

This poster outlines the history of math circles, provides a brief sampler of math circle styles and approaches, and offers a list of resources for learning more about math circles and finding support to start one of your own. Additional information, of course, can be found at the SIGMAA website http://sigmaa.maa.org/mcst, at the National Association of Math Circles, http://www.mathcircles.org, and at the Math Teachers’ Circle Network, http://www.mathteacherscircle.org. Please join the SIGMAA on Circles by adding the SIGMAA when you renew your membership with the MAA

Table 1a: Organization: National Association of Math Circles http://www.mathcircles.org/ Help for Student and Teacher Circles

Activity: Information Table

Presenters: Brandy Wiegers --brandy@msri.org --Math Science Research Institute

Amanda Serenevy --viajera6@gmail.com --Riverbend Community Math Center

MSRI Director of Educational and Outreach Activities: Alissa Crans --acrans@msri.org --Math Science Research Institute

Organization Description: The National Association of Math Circles, at the Mathematical Sciences Research Institute, runs the mathcircles.org website which provides a central resource for people wishing to start new math circles or sustain existing ones. For new math circle leaders, the NAMC provides a comprehensive guide to math circles, the Circle In a Box book, and a useful wiki. The site includes links to dozens of math circles all over the country as well as some international circles, a problem database and lesson plan collection, videos of math circle sessions, information about math circle minigrants, and descriptions of math circle events at national math meetings as well as at the annual Circle on the Road conference. Whether your circle is new or has been running for years, please register for an account at http://www.mathcircles.org/, add your circle to our list, and apply for a minigrant!

Table 1b: Presentation Times: 2:30 3:00 Organization: San Francisco Math Circle --http://www.sfmathcircle.org/index.html/ --Student & Teachers, all grades

Activity: Keeping Safe: Lessons Learned Working with SFMC Elementary Students

Presenter: Brandy Wiegers brandy@msri.org --Math Science Research Institute

Organization Description: SFMC is a weekly program for teachers and students centering around a community of students who want to work together on intriguing and challenging mathematical problems. We are excited to add a new program to San Francisco Math Circle (SFMC) aimed at students in grades 2, 3, 4 and 5. The program, SFMC Elementary, is designed to develop a positive attitude towards mathematics by introducing young children to elements of mathematical culture. It has the same mission and philosophy as the SFMC.

Activity Description: We have learned a lot about best practices in working with elementary students to keep everyone safe over the last year. I would like to use my activity session to share some of these lessons and talk with anyone who might have questions about their own location. I would also share a few of our favorite activities.

Table 2a: Organization: Math Teachers' Circles Network --http://www.mathteacherscircle.org/ --Help for Teacher Circles

Presenters: Diana White --Diana.White@ucdenver.edu --University of Colorado Denver

Tatiana Shubin --tatiana.shubin@sjsu.edu --San Jose State University

Organization Description: Math Teachers' Circles (MTCs) are groups of teachers who meet regularly with mathematicians for highly interactive sessions focused on problem solving in the context of rich mathematics. The goal is to involve these teachers in the mathematical community by putting them in direct contact with mathematicians and engaging them in an authentic, ongoing mathematical experience that will ultimately impact their understanding and teaching of mathematics.

The MTC Network (http://www.mathteacherscircle.org/) is a project of the American Institute of Mathematics (AIM; www.aimath.org) that links together MTCs throughout the United States. To help the MTC community grow, the Network organizes two workshops on “How to Run a Math Teachers’ Circle” each summer and provides extensive mathematical and logistical resources to local MTCs. This poster gives an overview of MTCs and their outcomes, and describes the workshops and other resources offered by the MTC Network. Poster

Table 2b: Presentation Times: 2:00 3:30 Organization: Math Teachers' Circles Network --http://www.mathteacherscircle.org/ --Teacher Circles

Poster: Research Update on Math Teachers' Circles

Presenter: Diana White --Diana.White@ucdenver.edu --University of Colorado Denver

Poster Description: This poster/activity will update the national Math Circle community on the status of the research related to Math Teachers' Circles as part of an NSF DRK12 grant.

Table 3: Presentation Times: 1:30 2:30 Organization: Navajo Nation Math Circles Project no web site – Students and Teachers

Activity: Navajo-Related Math Circle Activities

Presenters: Tatiana Shubin --tatiana.shubin@sjsu.edu --San Jose State University

Henry Fowler --tatiana.shubin@sjsu.edu--Dine College, Tsaile

Organization Description: The Navajo Nation Math Circles Project (NNMCP) is an initiative to launch and sustain math circles for students and teachers on the Navajo Reservation. The project began with first circles started in September, 2012, at two locations -St Michael Indian School and Chinle High School, and a math circle-style sessions conducted for pre-service teachers at the Dine College in Tsaile. The project is supported by NSF, AIM, MSRI, and EAF.

Activity Description: The activities reflect the highly visual nature of Navajo kids' learning style and their tendency to be reserved yet cooperative.

Table 4: Presentation Times: 1:00 2:00 Organization: Riverbend Community Math Center --http://riverbendmath.org/ --Student and Teacher Program

Activity: Exploring Lill's Method for Finding Polynomial Roots

Presenters: Amanda Serenevy --viajera6@gmail.com --Riverbend Community Math Center

Organization Description:

The Riverbend Community Math Center is a non-profit organization located in South Bend, Indiana that started offering programs during the fall of 2006. Our mission is to promote interest and confidence in mathematics among people of all ages. Our organization provides several different types of programs including a Math and Technology Academy, a Math Studio, Math Circles, professional development workshops for teachers, and custom events with hands-on activities.

Activity Description:

Lill's method is a visual method for finding roots of a polynomial of any degree, and was developed in 1867 by Austrian engineer Eduard Lill. This method was used by Margharita Beloch in 1936 to solve cubic equations via origami constructions. During this Math Circle session, we will first play with Lill's method to see how it works in quadratic and cubic cases, and then extend the method to polynomials of higher degree. We will follow the presentation in the following reference: Thomas C. Hull (April 2011). "Solving Cubics With Creases: The Work of Beloch and Lill", American Mathematical Monthly: 307-315. This circle could work for students or teachers who are familiar with quadratics and polynomials. It works especially well for students in intermediate algebra, pre-calculus, and calculus courses, or for teachers who teach these courses.

Table 5: Presentation Times: 1:00 3:00 Organization: Albuquerque Math Teachers' Circle --http://www.unm.edu/~mathtc--Teacher Circle

Activity: What is in that Can of Soda?

Presenter: Michael Nakamaye --nakamaye@gmail.com --University of New Mexico

Organization Description: The Albuquerque Math teachers' circle started up two years ago. While our target audience is middle school teachers, we have several elementary school teachers who are regular participants and a couple of high school teachers as well. Most of our participants come from the very large Albuquerque Public School system but we frequently have teachers from private schools or from the suburbs in attendance. We have 6 meetings a year during the school year and have also had two summer workshops. Right now we are looking to build a core group of teachers who will eventually determine what direction our circle takes.

Activity Description: Participants will be presented with a can of soda and asked how they might go about determining how thick the can is. Cutting the can open and measuring is the most straightforward approach but it is dangerous because the can is quite sharp. The average thickness can be estimated indirectly, however, by approximating the surface area of the can (a good geometry exercise) and then using the density of aluminum. Alternatively, it is possible to find the volume of aluminum in the can directly using an idea of Archimedes: crush the can, submerge it in water, and measure the amount of water displaced. Once this is known, the thickness can again be approximated by dividing the volume by the surface area. The activity is geometrically rich while dealing with a simple every day object. For the Common Core - Archimedes and the King's Crown and Notes on Can Thickness Calulations

Table 6: Presentation Times: 2:30 3:30

Organization: University of Wisconsin Milwaukee (UWM) Math Circle -https://pantherfile.uwm.edu/gapinter/www/mc_index.html --Student Circle

Activity: Operation Cookie Jar

Presenter: Gabriella Pinter --gapinter@uwm.edu--University of Wisconsin, Milwaukee

Authors: Klara Pinter --University of Szeged and Istvan Lauko --University of Wisconsin

Organization Description: Our UWM Math Circle started in September 2011, and is led by three UWM Mathematics faculty, Gabriella Pinter, Chris Hruska and Boris Okun. We focus on open ended problem solving activities for students in grades 7-12. Our goal is to stimulate discussion, and to support the students in further explorations of problems. We emphasize rigorous arguments once the idea of a solution is outlined. We encourage students to ask new questions, and formulate new problems. Our Math Circle is free and students can join any time.

Activity Description: There are 15 cookie jars, numbered consecutively from 1 to 15. The number of cookies in each jar is equal to the number of the jar. A “move” consists of choosing one or more jars, then removing one or more cookies from the chosen jars—but the same number of cookies from each jar. Your task is to work out how to get all the cookies from all the jars in the smallest number of moves. (from The Inquisitive Problem Solver by P. Vaderlind, R. Guy and L. Larson, MAA, 2002. P34. Page 7.)

1. Discussion of approaches; Different representations, different ways of emptying jars

2. Problem solving strategy: try a simpler problem with smaller numbers (manipulate actual jars with tokens)

3. Recognize a pattern, formulate strategies of emptying jars (e.g., ‘take the most cookies’, empty the most jars’, ‘binary algorithm’)

4. Formulate conjecture, and prove. Generalization for n jars with 1,2,3,…,n cookies.

5. New questions:

(a) What if the jars contain cookies: a, a+1,a+2 ? Two or three steps?

(b) What if we consider a, a+d, a+2d, …, a+(n-1)d ? (n jars, number of cookies forming an arithmetic sequence – which strategy gives optimal results?)

(c) How to fill three jars so that 1,2 or 3 steps will be needed to empty them? What about four jars? Can n jars be filled in a way that n steps are needed to empty them? (Interesting fact: Jars with {1 2, 4, 8, 16} does not require 5 steps if negative steps (i.e., adding cookies) are also allowed: steps -5, 1, 7 and 9 would empty the jars, but here the order of steps would matter, while it did not matter in the original problem.) The discussion can be steered in different directions based on responses and ideas from the audience and can fill a whole hour or even more.

6. The original problem is interesting, because it is an intriguing ‘roots to research’ problem. The idea of finding an optimal subset representation for an arbitrary set of positive integers has attracted some attention in recent years. The abstract problem has consequences for finding approximation algorithms for minimizing segments in intensity modulated radiation therapy. In fact, a simple demonstration could be devised to illustrate the practical problem, and show its connection to the ‘cookie jar’.

Table 7: Presentation Times: 1:30 3:30

Organization:

Fairfield County Math Teachers’ Circle http://www.sacredheart.edu/academics/collegeofartssciences/academicdepartments/mathematics/fairfieldcountymathteacherscircle/ Middle School Teachers’ Circle

Activity: Pool Table Geometry

Presenters: Hema Gopalakrishnan GopalakrishnanH@sacredheart.edu --Sacred Heart University

Stephanie Furman --sfurman@darienps.org --Darien Public School, Darien CT

Organization Description: The Fairfield County Math Teachers’ Circle is a newly formed teachers’ circle that held its first summer immersion workshop in July 2012. Seventeen middle school math teachers enthusiastically participated in problem solving during the workshop. They were very grateful for the opportunity and expressed that the workshop did not compare to any class that they have taken. The pool table activity is one of the many successful activities at the summer workshop. Participants of the summer workshop are eager to return to Sacred Heart University for the six academic year meetings during the 2012 – 2013 school year.

Activity Description: This is a fun activity that examines the path of a ball on a pool table with pockets only at the four corners of the table. The ball starts at the bottom left corner at a 45 degree angle. Students can consider pool tables of different dimensions, draw pictures, gather data and find patterns. They can explain their observations and answer several questions using concepts from number theory and geometry learned in elementary and middle school.

Table 8: Presentation Times: 2:00 3:00 Organization: Richmond Math Salon --http://mathmamawrites.blogspot.com – Students, Teachers, Parents

Activity: What's Up With Spot It?

Presenter: Sue VanHattum --mathanthologyeditor@gmail.com --Contra Costa College

Organization Description:

The informal math party held at my home can be seen here:

http://mathmamawrites.blogspot.com/2010/08/richmond-math-salon-sweet-sampling_08.html

We used to meet monthly; now we meet once in the fall and once in the spring. It's a whole family event. The parents want to provide math enrichment for their kids, and I want to get the parents pulled in too.

Activity Description:

The game of Spot It has 55 cards with 8 pictures on each card. The object of the game is to find a match between your card and the center card before your opponents do. It's a fun game to play in a group of adults and kids -sometimes the youngest ones win. There's no math in the playing of this game, but you might have some math questions after you play it. We'll play a few rounds, talk about the questions participants have, and then think about those questions together.

Table 9: Presentation Times: 1:00 2:30 Organizations: Math Teachers’ Circle of Austin --https://sites.google.com/site/mtcaustin/ --Middle School Teachers’ Circle

Activity: Folding Polygons

Presenters: Altha B. Rodin --rodin@math.utexas.edu --University of Texas at Austin

Organization Description: The Math Teachers’ Circle of Austin has been in operation since the summer of 2010 when we held our first Summer Immersion Workshop. The founding members of the MTCA are Altha Rodin and Adriana Sofer, faculty members in the math department of The University of Texas, Jason Ermer, who is part of the UTeach program, and Patty Hill and Michael Word, teachers at the Kealing Middle School Magnet program. We have been joined by two new members of the mathematics department, Zachary Miner and Cristina Caputo. We are fortunate to have a vibrant group of middle school math teachers in the Austin area who regularly attend our problem solving sessions. For more information, please visit our web site: http://sites.google.com/site/mtcaustin/

Activity Description: One may take a long, thin strip of paper, fold it up then unfold it to see the angle formed by the crease line and the bottom edge of the paper. The paper can then be folded down so that the top edge of the paper falls along the first crease line. If one continues folding in this way, alternating folding up and down along the crease lines, the angles formed by the crease lines and the edge of the paper stabilize and the triangles that appear seem to be equilateral. If the first few triangles are cut off and thrown away, the resulting strip of paper can be folded to form a hexagon. We will see why the angles stabilize and will investigate other folding sequences to determine what angles they produce and which polygons can be folded from the resulting strip of paper.

Table 10: Presentation Times: 1:30 2:00 Organization: Texas A&M Math Circle --http://mathcircle.tamu.edu --Middle School Student Circle

Activity: Hyperbolic Soccer Ball

Presenter: Frank Sottile --sottile@math.tamu.edu --Texas A&M University

Organization Description: The TAMU Math Circle meets weekly at Texas A&M University each Saturday for students in grades 5-8. It is organized by Sottile and Phil Yasskin of the Mathematics Department and Alex Sprintson of the Electrical and Computer Engineering Department at Texas A&M, and supported by parent volunteers, graduate students, and postdocs. Each meeting begins with a half hour of unstructured mathematical activity (games, puzzles, or mathematical toys) after which the students split into two groups for a 90 minute structured activity (including a snack break). For more, see its web page: http://mathcircle.tamu.edu

Activity Description: I will present the activity "Hyperbolic Soccer Ball". This is suitable for all ages, having been tested on middle school students, on undergraduates, and on college teachers in Nigeria. It illustrates key geometric features of the hyperbolic plane, and the participants create a beautiful and thought-provoking mathematical model. (See more details here)

Table 11: Presentation Times: 1:30 3:00 Organization: SIGMAA-MCST --http://sigmaa.maa.org/mcst/ --Help for Student and Teacher

Poster: Math Circles and the Common Core State Standards

Presenters: Amanda Serenevy --viajera6@gmail.com --Riverbend Community Math Center

James Tanton --jamestanton@stmarksschool.org --St Mark's School

Poster Description: The Common Core State Standards are a set of math content and practice guidelines for K-12 math education in most states. States are ramping up towards implementation now, with full transition to the standards at every grade level slated for 2014. The Common Core State Standards present an opportunity and a challenge. These standards oblige all teachers to implement mathematics curricula grounded in conceptual understanding, higher level critical thinking, and mathematical modeling. While previous state standards were written with the intention that good teachers would incorporate these aspects of instruction, the Common Core State Standards make these requirements more explicit. Effective implementation of the Common Core State Standards would radically improve math instruction in the United States. However, the change from past modes of instruction is significant, and teachers will need support to make the transition from the instructional methods they have always known. The depth of content knowledge required is also much greater, especially for 3rd through 8th grade teachers, and teachers are under a great deal of pressure to learn the content they need to know and to find curricula they can use. Mathematicians can help by assisting local teachers with additional training in content knowledge, by carefully reevaluating the training of future teachers conducted under the auspices of the math department or in collaboration with schools of education, and by helping to identify high quality topics/activities/curricula which teachers could use in their classrooms. At this poster, we invite participants to look at the principal content topics in the 6th and 7th grade standards to brainstorm ideas for great related Math Circle topics. Please stop by to add your ideas to the list!